Magic squares have fascinated mathematicians for centuries and they continue to do so today. However, many questions remain unanswered, and this study proposes a different perspective in order to shed new light on what magic squares are and how they work. Considering magic squares to be flattened partial viewpoints of convex or concave magic tori, the implied Gaussian surfaces require a modular arithmetic approach that is tested and analysed here.
Until today, most magic square construction methods use base squares, whilst "Magic Torus Coordinate and Vector Symmetries" proposes modular coordinate equations that not only define specific magic tori, but also generate their magic torus descendants.
The construction of Agrippa's traditional magic squares is analysed in detail for each of the seven planetary magic tori, and modular coordinate equations are defined that generate descendant tori throughout the respective higher-orders, whether they be odd, doubly-even, or singly-even.
The unique third-order magic torus and also a fifth-order pandiagonal torus of Latin construction are examined in detail. The modular coordinate equations that are defined generate an interesting variety of higher-odd-order descendant tori.
The study also explores the construction of the 12 different types of fourth-order magic tori, as well as the generation of their doubly-even magic torus descendants. In addition, a fourth-order Candy-style perfect square pandiagonal torus, and a fourth-order unidirectionally pandiagonal semi-magic torus, are each analysed in detail, and their torus descendants generated, throughout their respective higher-orders.
At the end of the study observations are made, and some conclusions are drawn as to the signification of the findings, and the potential for future research.
Some sample illustrations of a fourth-order partially pandiagonal torus and its partially pandiagonal torus descendants are shown below:
 |
| T4.195 4th-order partially pandiagonal torus |
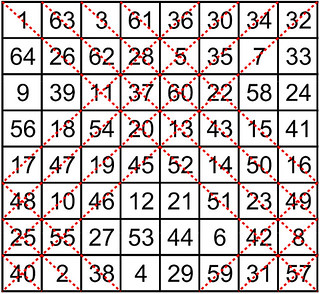 |
| 8th-order partially pandiagonal torus, direct descendant of T4.195 |
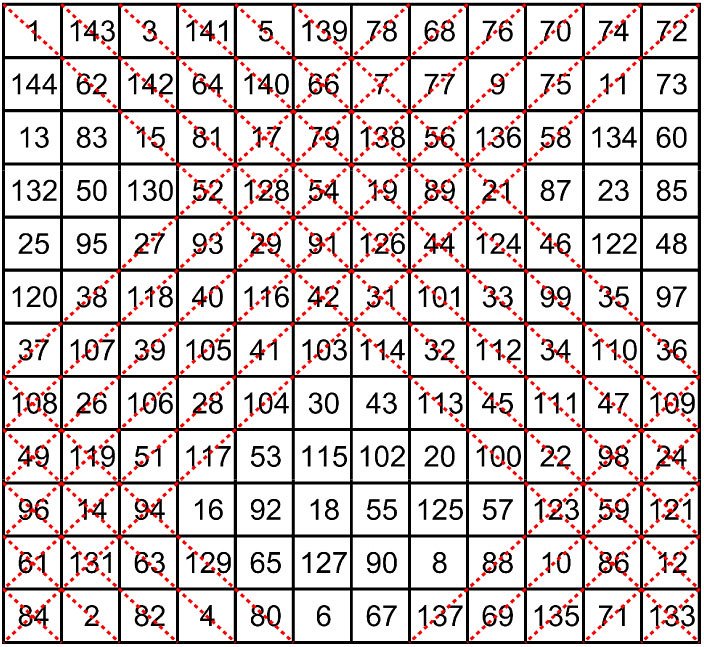 |
| 12th-order partially pandiagonal torus, direct descendant of T4.195 |
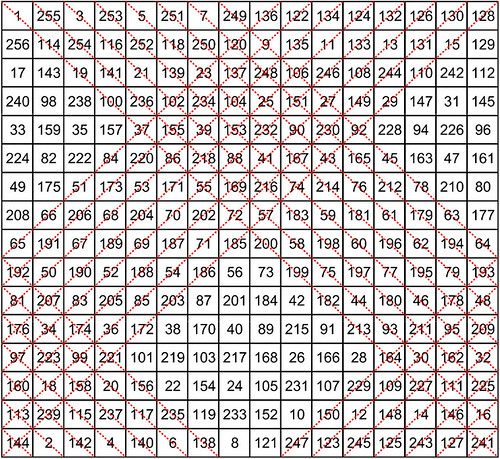 |
| 16th-order partially pandiagonal torus, direct descendant of T4.195 |
To download "Magic Torus Coordinate and Vector Symmetries" from Google Drive, please use the following link: MTCVS 161019. The 134 Mo pdf file exceeds the maximum size (25 Mo) that Google Drive can scan, but as the file is virus free you can download it safely. Depending on the speed of your computer, the download can take up to two or three minutes to complete.
