After my previous post "Area Magic Squares and Tori of Order-3," Walter Trump continued his computer research of the linear area magic squares of order-4, now joined in this venture by a fellow mathematician and programmer Hans-Bernhard Meyer.
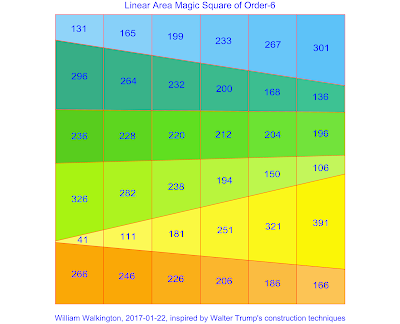
Having already some insight into the linear area magic squares of the odd-order-3 and of the doubly-even-order-4, it was tempting to search for a singly-even-order example. I therefore decided to do some exploring, and on the 22nd January 2017, I found this first linear area magic square of order-6:
For the area magic square shown above, the magic constant of each row, column and main diagonal is 1296 (64). The total areas are therefore 7776 (65). The mean number of each row, column and main diagonal is 216 (63). The last digits of the first and last numbers of the horizontal non-parallel rows are either 1 for odd number sequences, or 6 for even number sequences. Please note that the area cell 41 is not a triangle, but a trapezium (or trapezoid) like all the other cells. For those amongst you who may be interested in the mathematical construction, Hans-Bernhard Meyer has since very kindly produced the following parameterisation:
From then on using a computer program to find many more examples, on the 26th January 2017, Hans-Bernhard Meyer drew to my attention a new linear area magic square with not only parallel vertical lines, but also a central horizontal line. He kindly authorised me to publish this example illustrated below:
The magic constant of this linear area magic square (L-AMS) is 474, and the total areas are 2,844. The last digits of the first and last numbers of the non-parallel rows are either 4 for the even number sequences, or 9 for the odd number sequences. I noticed that in addition to the central horizontal line, there was also a pair of broken magic diagonals which would enable an easy transformation, as illustrated below:
It can be seen that these are two essentially different L-AMS viewpoints of a same area magic cylinder. Please note that there are also another four area semi-magic parallelograms displayed on this cylinder (with cells 44, 124, 144, or 19 in their top left corners).
On the 29th January 2017, Hans-Bernhard Meyer informed me that the following can be proved:
Every 6x6 L-AMS with magic sum S and horizontal centre line has the property:
x04+x11+x18+x19+x26+x33 = x03+x08+x13+x24+x29+x34 = S
and conversely, every 6x6 L-AMS with magic sum S and
x04+x11+x18+x19+x26+x33 = x03+x08+x13+x24+x29+x34 = S
has a horizontal centre line.
He stated that there are many examples of such L-AMS and strongly supposed that the example illustrated above had the lowest possible magic sum. He was able to confirm that examples with 2 horizontal lines do not exist.
On the same day, Hans-Bernhard also informed me that in the order-6, the L-AMS with the lowest possible magic sum had a magic constant of 402. He had found that there were several of these L-AMS, and kindly sent me details of an example, which I have illustrated below:
On the 29th January 2017, Hans-Bernhard Meyer informed me that the following can be proved:
Every 6x6 L-AMS with magic sum S and horizontal centre line has the property:
x04+x11+x18+x19+x26+x33 = x03+x08+x13+x24+x29+x34 = S
and conversely, every 6x6 L-AMS with magic sum S and
x04+x11+x18+x19+x26+x33 = x03+x08+x13+x24+x29+x34 = S
has a horizontal centre line.
He stated that there are many examples of such L-AMS and strongly supposed that the example illustrated above had the lowest possible magic sum. He was able to confirm that examples with 2 horizontal lines do not exist.
On the same day, Hans-Bernhard also informed me that in the order-6, the L-AMS with the lowest possible magic sum had a magic constant of 402. He had found that there were several of these L-AMS, and kindly sent me details of an example, which I have illustrated below:
The total areas of this square are 2,412. The last digits of the first and last numbers of the non-parallel rows are either 2 for the even number sequences, or 7 for the odd number sequences. The even number sequence of the bottom row begins with 12, which Hans-Bernhard informs me is the lowest possible vertex area for L-AMS (linear area magic squares) of order-6.
These are only the first of an infinite number of examples that remain to be discovered, and I therefore expect to be regularly publishing updates to this post!
A former post Area Magic Squares and Tori of Order-3 can be found in these pages since the 13th January 2017.
On the 25th January 2017, Hans-Bernhard Meyer published an article entitled Observations on 4x4 Area Magic Squares with vertical lines in his website: Math'-pages.
On the 3rd February 2017, Walter Trump published a chapter entitled Area Magic Squares in his website: Notes on Magic Squares. This chapter includes many analyses and examples of area magic squares of the third and fourth-orders.
Since the 8th February 2017, "Area Magic Squares of Order-4" relates the first findings of area magic squares of the fourth-order.
In the N° 487 2018 May issue of "Pour La Science" (the French edition of Scientific American), Professor Jean-Paul Delahaye has written an article entitled "Les Carrés Magiques d'Aires."
In the December 2018 issue of "Spektrum der Wissenschaft" (a Springer Nature journal, and the German edition of Scientific American), Professor Jean-Paul Delahaye has written an article entitled "FLÄCHENMAGISCHE QUADRATE."
Related links
A former post Area Magic Squares and Tori of Order-3 can be found in these pages since the 13th January 2017.
On the 25th January 2017, Hans-Bernhard Meyer published an article entitled Observations on 4x4 Area Magic Squares with vertical lines in his website: Math'-pages.
On the 3rd February 2017, Walter Trump published a chapter entitled Area Magic Squares in his website: Notes on Magic Squares. This chapter includes many analyses and examples of area magic squares of the third and fourth-orders.
Since the 8th February 2017, "Area Magic Squares of Order-4" relates the first findings of area magic squares of the fourth-order.
In the N° 487 2018 May issue of "Pour La Science" (the French edition of Scientific American), Professor Jean-Paul Delahaye has written an article entitled "Les Carrés Magiques d'Aires."
In the December 2018 issue of "Spektrum der Wissenschaft" (a Springer Nature journal, and the German edition of Scientific American), Professor Jean-Paul Delahaye has written an article entitled "FLÄCHENMAGISCHE QUADRATE."
On the 21st May 2021, Yoshiaki Araki (面積魔方陣がテセレーションみたいな件
@alytile) tweeted several order-4 and order-3 solutions in a polyomino area magic square thread.
These included (amongst others) an order-4 example constructed with 16
assemblies of 5 to 20 dominoes. For more information on polyomino area
magic squares please check the links at the end of the article "Area Magic Squares of Order-3."
Since the 22nd June 2021, Inder Taneja has published a paper entitled "Creative Magic Squares: Area Representations" in which he explores polyomino area magic using perfect square magic sums.
A new post on "Polyomino Area Magic Tori" can be found in these pages since the 7th June 2022.






No comments:
Post a Comment
Or, should you prefer to send a private message, please email william.walkington@wandoo.fr