Sub-Magic 2x2 Squares on Fourth-Order Magic Tori

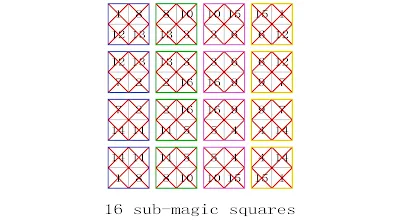
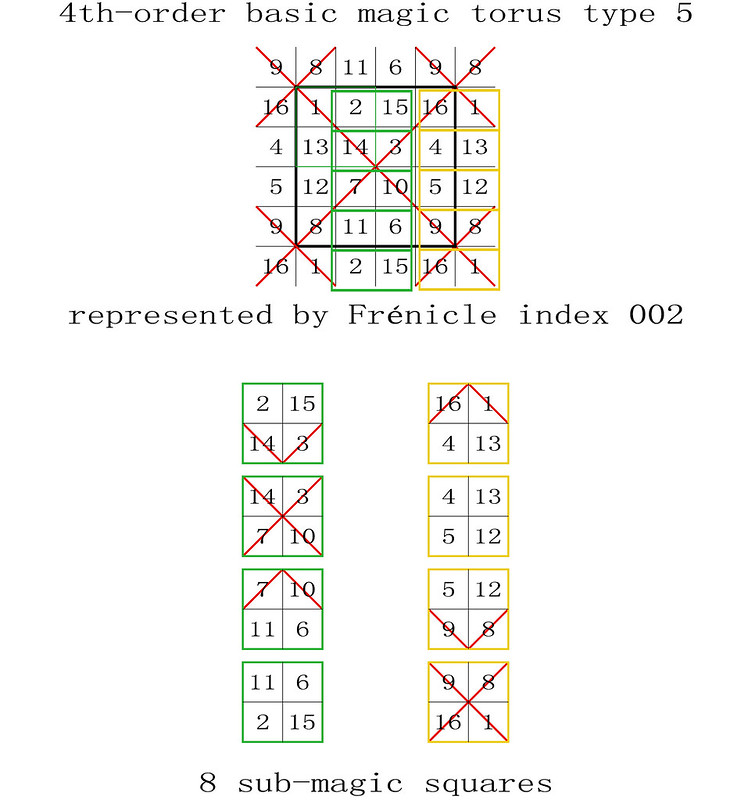
My recent studies have shown that 2x2 sub-magic squares are fundamental in the way the fourth-order magic tori work.

It has long been known that fourth-order magic squares have 2x2 sub-magic squares at their centres and that the 4 corner numbers of the squares also add up to 34. However, once considered as magic tori, the 4 corner numbers of each 4th-order magic square are in fact another sub-magic 2x2 square, and more subsquares are to be found overlapping the former boundary of the initial square.

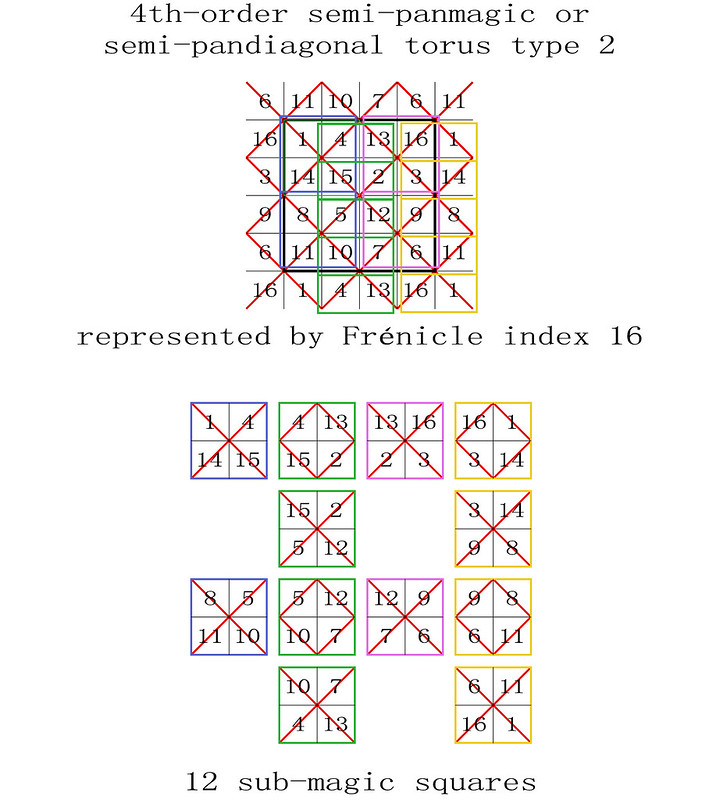
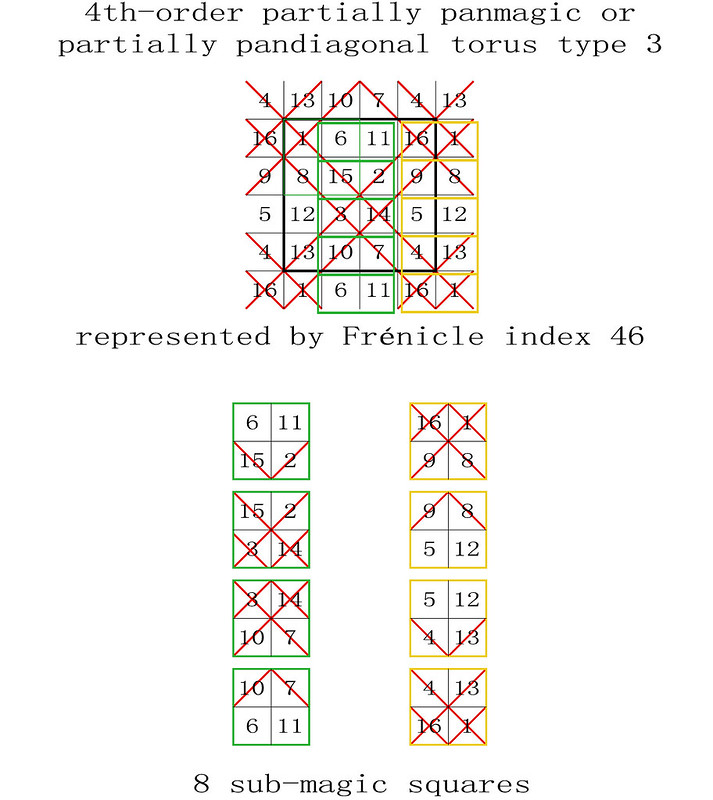
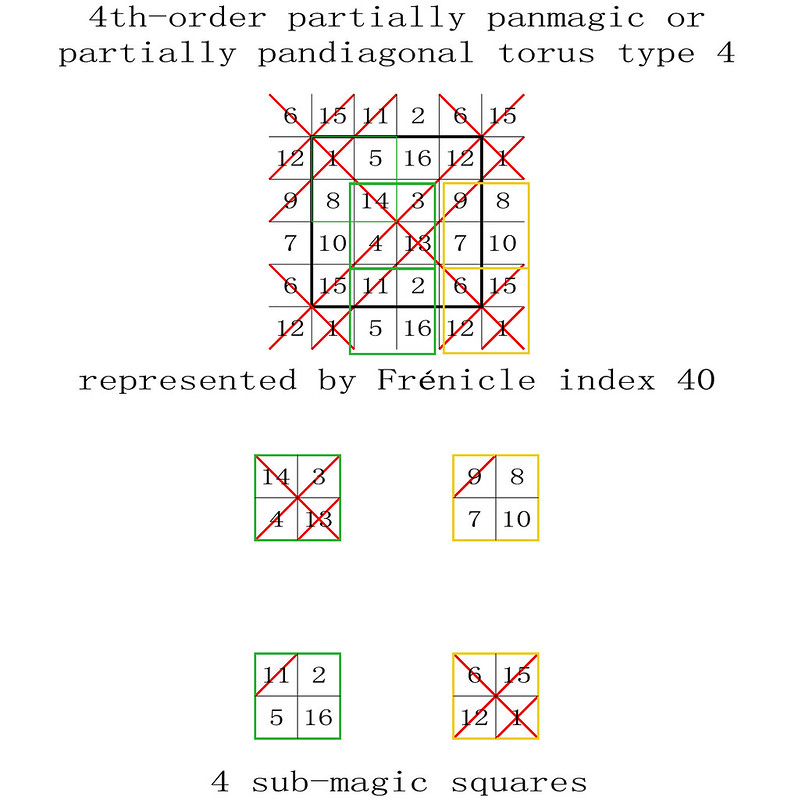
I have therefore tried to determine the number of magic sub-magic squares that are displayed on each type of the fourth-order magic tori, and my first results are illustrated below. Using simple observation and manual input I have not verified all of the 880 Frénicle fourth-order squares and may have missed something. Your comments and corrections are welcome.

Surprisingly the fourth-order type 4 partially panmagic torus displays less sub-magic 2x2 squares than the basic fourth-order type 5 magic torus.

Hypothesis

Each number of a fourth-order magic torus (or magic square) comes from at least one 2x2 sub-magic square. Or, expressed otherwise, the surface of every fourth-order magic torus is entirely covered by 2x2 sub-magic squares.

Conclusion

The above remained to be verified by a computer programme until the day after this article was published when Harry White very kindly checked and validated the hypothesis: His results confirm that every 4th-order magic torus (or magic square) is entirely covered by 2x2 sub-magic squares. For all the 880 Frénicle squares he has found 7,712 sub-squares and has confirmed that all the numbers of the different magic squares come from at least one 2x2 sub-magic square.

Harry White's results show that within a same type of tori the patterns and numbers of sub-squares can vary. For example, some of the type 5 tori have a reduced cover of 4 sub-magic squares instead of the 8 subsquares shown above (for example the Frénicle square index 277). This will need to be investigated further.

Please note that I have just published (the 28th March 2013) a new article that takes into account the different arrangements of the sub-magic 2x2 squares, and which attempts to complete the picture.

As for 4th-order semi-magic squares I have started to look into the question and have discovered some examples which are totally covered by 4 2x2 sub-magic squares whilst other examples have no subsquares at all: The phenomenon is not just limited to the magic tori.